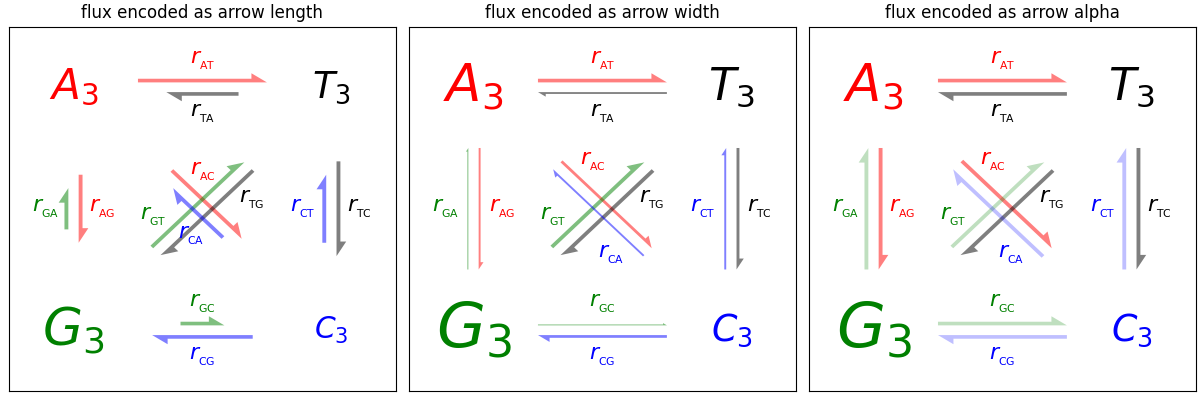
Flèche Démo

Trois façons de dessiner des flèches pour coder la "force" des flèches (par exemple, les probabilités de transition dans un modèle de Markov) en utilisant la longueur, la largeur ou l'alpha (opacité) des flèches.

import itertools

import matplotlib.pyplot as plt
import numpy as np


def make_arrow_graph(ax, data, size=4, display='length', shape='right',
                     max_arrow_width=0.03, arrow_sep=0.02, alpha=0.5,
                     normalize_data=False, ec=None, labelcolor=None,
                     **kwargs):
    """
    Makes an arrow plot.

    Parameters
    ----------
    ax
        The axes where the graph is drawn.
    data
        Dict with probabilities for the bases and pair transitions.
    size
        Size of the plot, in inches.
    display : {'length', 'width', 'alpha'}
        The arrow property to change.
    shape : {'full', 'left', 'right'}
        For full or half arrows.
    max_arrow_width : float
        Maximum width of an arrow, in data coordinates.
    arrow_sep : float
        Separation between arrows in a pair, in data coordinates.
    alpha : float
        Maximum opacity of arrows.
    **kwargs
        `.FancyArrow` properties, e.g. *linewidth* or *edgecolor*.
    """

    ax.set(xlim=(-0.25, 1.25), ylim=(-0.25, 1.25), xticks=[], yticks=[],
           title=f'flux encoded as arrow {display}')
    max_text_size = size * 12
    min_text_size = size
    label_text_size = size * 4

    bases = 'ATGC'
    coords = {
        'A': np.array([0, 1]),
        'T': np.array([1, 1]),
        'G': np.array([0, 0]),
        'C': np.array([1, 0]),
    }
    colors = {'A': 'r', 'T': 'k', 'G': 'g', 'C': 'b'}

    for base in bases:
        fontsize = np.clip(max_text_size * data[base]**(1/2),
                           min_text_size, max_text_size)
        ax.text(*coords[base], f'${base}_3$',
                color=colors[base], size=fontsize,
                horizontalalignment='center', verticalalignment='center',
                weight='bold')

    arrow_h_offset = 0.25  # data coordinates, empirically determined
    max_arrow_length = 1 - 2 * arrow_h_offset
    max_head_width = 2.5 * max_arrow_width
    max_head_length = 2 * max_arrow_width
    sf = 0.6  # max arrow size represents this in data coords

    if normalize_data:
        # find maximum value for rates, i.e. where keys are 2 chars long
        max_val = max((v for k, v in data.items() if len(k) == 2), default=0)
        # divide rates by max val, multiply by arrow scale factor
        for k, v in data.items():
            data[k] = v / max_val * sf

    # iterate over strings 'AT', 'TA', 'AG', 'GA', etc.
    for pair in map(''.join, itertools.permutations(bases, 2)):
        # set the length of the arrow
        if display == 'length':
            length = (max_head_length
                      + data[pair] / sf * (max_arrow_length - max_head_length))
        else:
            length = max_arrow_length
        # set the transparency of the arrow
        if display == 'alpha':
            alpha = min(data[pair] / sf, alpha)
        # set the width of the arrow
        if display == 'width':
            scale = data[pair] / sf
            width = max_arrow_width * scale
            head_width = max_head_width * scale
            head_length = max_head_length * scale
        else:
            width = max_arrow_width
            head_width = max_head_width
            head_length = max_head_length

        fc = colors[pair[0]]

        cp0 = coords[pair[0]]
        cp1 = coords[pair[1]]
        # unit vector in arrow direction
        delta = cos, sin = (cp1 - cp0) / np.hypot(*(cp1 - cp0))
        x_pos, y_pos = (
            (cp0 + cp1) / 2  # midpoint
            - delta * length / 2  # half the arrow length
            + np.array([-sin, cos]) * arrow_sep  # shift outwards by arrow_sep
        )
        ax.arrow(
            x_pos, y_pos, cos * length, sin * length,
            fc=fc, ec=ec or fc, alpha=alpha, width=width,
            head_width=head_width, head_length=head_length, shape=shape,
            length_includes_head=True,
            **kwargs
        )

        # figure out coordinates for text:
        # if drawing relative to base: x and y are same as for arrow
        # dx and dy are one arrow width left and up
        orig_positions = {
            'base': [3 * max_arrow_width, 3 * max_arrow_width],
            'center': [length / 2, 3 * max_arrow_width],
            'tip': [length - 3 * max_arrow_width, 3 * max_arrow_width],
        }
        # for diagonal arrows, put the label at the arrow base
        # for vertical or horizontal arrows, center the label
        where = 'base' if (cp0 != cp1).all() else 'center'
        # rotate based on direction of arrow (cos, sin)
        M = [[cos, -sin], [sin, cos]]
        x, y = np.dot(M, orig_positions[where]) + [x_pos, y_pos]
        label = r'$r_{_{\mathrm{%s}}}$' % (pair,)
        ax.text(x, y, label, size=label_text_size, ha='center', va='center',
                color=labelcolor or fc)


if __name__ == '__main__':
    data = {  # test data
        'A': 0.4, 'T': 0.3, 'G': 0.6, 'C': 0.2,
        'AT': 0.4, 'AC': 0.3, 'AG': 0.2,
        'TA': 0.2, 'TC': 0.3, 'TG': 0.4,
        'CT': 0.2, 'CG': 0.3, 'CA': 0.2,
        'GA': 0.1, 'GT': 0.4, 'GC': 0.1,
    }

    size = 4
    fig = plt.figure(figsize=(3 * size, size), constrained_layout=True)
    axs = fig.subplot_mosaic([["length", "width", "alpha"]])

    for display, ax in axs.items():
        make_arrow_graph(
            ax, data, display=display, linewidth=0.001, edgecolor=None,
            normalize_data=True, size=size)

    plt.show()